3.7 Continuity And Differentiablityap Calculus Algebra
Calculus Introduction: Continuity and Differentiability Notes, Examples, and Practice Quiz (w/solutions) Topics include definition of continuous, limits and asymptotes, differentiable function, and more. Worksheet 3:7 Continuity and Limits Section 1Limits Limits were mentioned without very much explanation in the previous worksheet. We will now take a closer look at limits and, in particular, the limits of functions. Limits are very important in maths, but more specically in calculus.
 Show All NotesHide All Notes
Show All NotesHide All NotesSection 2-9 : Continuity
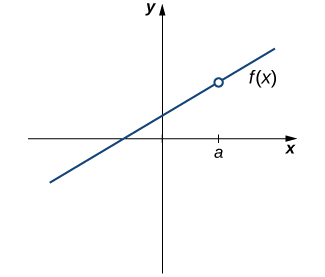
- The graph of (fleft( x right)) is given below. Based on this graph determine where the function is discontinuous. Solution
- The graph of (fleft( x right)) is given below. Based on this graph determine where the function is discontinuous. Solution
For problems 3 – 7 using only Properties 1 – 9 from the Limit Properties section, one-sided limit properties (if needed) and the definition of continuity determine if the given function is continuous or discontinuous at the indicated points.
- (displaystyle fleft( x right) = frac{{4x + 5}}{{9 - 3x}})
- (x = - 1)
- (x = 0)
- (x = 3)
- (displaystyle gleft( z right) = frac{6}{{{z^2} - 3z - 10}})
- (z = - 2)
- (z = 0)
- (z = 5)
- (gleft( x right) = left{ {begin{array}{rl}{2x}&{x < 6}{x - 1}&{x ge 6}end{array}} right.)
- (x = 4)
- (x = 6)
- (hleft( t right) = left{ {begin{array}{rl}{{t^2}}&{t < - 2}{t + 6}&{t ge - 2}end{array}} right.)
- (t = - 2)
- (t = 10)
- (gleft( x right) = left{ {begin{array}{rc}{1 - 3x}&{x < - 6}7&{x = - 6}{{x^3}}&{ - 6 < x < 1}1&{x = 1}{2 - x}&{x > 1}end{array}} right.)
- (x = - 6)
- (x = 1)
For problems 8 – 12 determine where the given function is discontinuous.
3.7 Continuity And Differentiablityap Calculus Calculator
- (displaystyle fleft( x right) = frac{{{x^2} - 9}}{{3{x^2} + 2x - 8}}) Solution
- (displaystyle Rleft( t right) = frac{{8t}}{{{t^2} - 9t - 1}}) Solution
- (displaystyle hleft( z right) = frac{1}{{2 - 4cos left( {3z} right)}}) Solution
- (displaystyle yleft( x right) = frac{x}{{7 - {{bf{e}}^{2x + 3}}}}) Solution
- (gleft( x right) = tan left( {2x} right)) Solution
3.7 Continuity And Differentiablityap Calculus Solver
For problems 13 – 15 use the Intermediate Value Theorem to show that the given equation has at least one solution in the indicated interval. Note that you are NOT asked to find the solution only show that at least one must exist in the indicated interval.
3.7 Continuity And Differentiablityap Calculus 14th Edition
- (25 - 8{x^2} - {x^3} = 0) on (left[ { - 2,4} right]) Solution
- ({w^2} - 4ln left( {5w + 2} right) = 0) on (left[ {0,4} right]) Solution
- (4t + 10{{bf{e}}^t} - {{bf{e}}^{2t}} = 0) on (left[ {1,3} right]) Solution
